Because the same mathematical principles can connect all these different experiences—both physical and psychological— Dr. Saniga asserts that any attempt to dismiss non-ordinary states of consciousness like NDEs “as pure hallucinatory phenomena would simultaneously cast a doubtful eye on the very role of mathematics in our understanding of Nature. To the contrary, it is mathematics itself...that tells us that it is far more natural to expect all these ‘unusual’ perceptions of time to be simply as real as our ordinary (‘normal’) one.”3
Dr. Saniga’s model cannot be fully described without using the specialized mathematics known as ‘algebraic projective geometry’. And since most of us have not been trained in that math, I am forced to risk oversimplifying his explanation a little bit here, in order to share it with the general public.
Recall how we all commonly think of time, as we go about our daily lives: time seems to be a simple ‘arrow’ or straight line running from the past, through the present, and pointing toward the future. However,
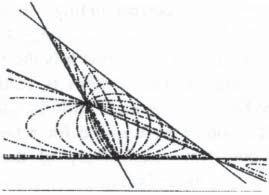 |
| Geometric representation of NDErs’ experience of time as “pure present” |
as Dr. Saniga points out, the fundamental equations of physics do not really insist on such a one-directional experience of time:
It is a well-known fact that the fundamental equations of physics are time-reversible, i.e. they do not distinguish between the past and future. Moreover, the very concept of the present, the now, has no proper place in the temporal of physics at all; this holds true whether one is talking of classical physics, quantum mechanics, or relativity theory.4
References
3 Metod Saniga, “Algebraic Geometry ...” Op. cit., page 159 [or page 23 inthe online form].
4 Ibid., page 137.
